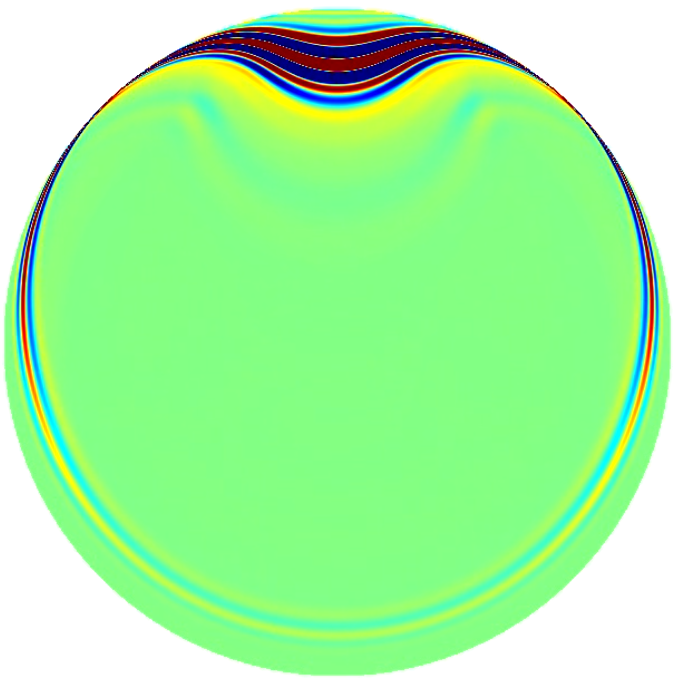
Helioseismology
Using these measurements, our aim in a collaboration with Laurent Gizon is to learn about the solar interior. To achieve this goal, we need to know how the quantities we want to learn about (velocity, sound speed, magnetic field, ...) are linked to the observations. This step is called the forward modeling.
Using these measurements, our aim in a collaboration with Laurent Gizon is to learn about the solar interior. To achieve this goal, we need to know how the quantities we want to learn about (velocity, sound speed, magnetic field, ...) are linked to the observations. This step is called the forward modeling.

Anwendungen